The goal is to ‘grow’ a mesh using DLA methods: a bunch of particles moving in a pseudo-random walk (biased to move down) fall towards a mesh. When a particle, which has an associated radius, intersects a vertex of the mesh that vertex moves outward in the normal direction by a small amount. Normal Direction means perpendicular to the surface (this is always an approximation for a mesh) Long edges get subdivided. Short edges get collapsed away. The results are rather spikey. It would be better if it was smoother. Or maybe it just needs to run longer.
Ideas for making smoother:
- have the neighbors of a growing vertex also grow, but by an amount proportional to the distance to the growing vertex. One could say the vertices share some nutrients in this scenario, or perhaps that a given particle is a sort of vague approximation of where some nutrients will land.
- let the particles have no radius. Instead have each vertex have an associated radius (a sphere around each vertex), which captures particles. This sphere could be at the vertex location, or offset a little ways away along the vertex normal. This sphere could be considered the ‘mouth’ of the vertex
- maybe let points fall on the mesh, find where those points intersect the mesh, and then grow the vertices nearby. Or perhaps vertices that share the intersected face.
Ideas for making Faster:
- Discretize space for moving particles about. This might require going back and forth between mesh and voxel space (discretized space is split up into ‘voxels’ – volumetric pixel)
- moving the spawning plane up as the mesh grows so that it can stay pretty close to the mesh
- more efficient testing for each particle’s relationship to the mesh (distance or something)
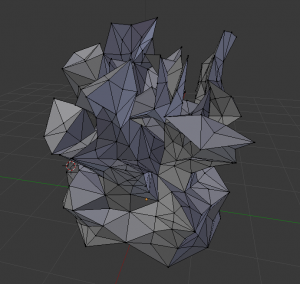
This one came out pretty branchy but its really jagged and the top branches get rather thin and platelike. This is why growing neighbor vertices (sharing nutrients) might be better.